A Practitioner’s Insights on Student Misconceptions and Errors
Does a bowling ball drop faster from the top of a building than a ping pong ball? Is it cheaper to rent a car from company A or company B? These are the kinds of questions students should be able to answer by applying knowledge gained in physics and mathematics lessons, yet many don’t fully understand the basic concepts.
The Trends in International Mathematics and Science Study (TIMSS) and TIMSS Advanced assessments are a rich source of data on student performance in these subjects in grades 4, 8, and 12. AIR researchers Teresa Neidorf, Alka Arora, Ebru Erberber, Yemurai Tsokodayi, and Thanh Mai methodically analyzed these data across multiple assessment cycles. By examining student performance on a set of assessment items, otherwise known as questions, they identified student understandings-and misunderstandings-related to two core concepts (gravity in physics and linear equations in mathematics).
The researchers’ open-access report, Student Misconceptions and Errors in Physics and Mathematics: Exploring Data from TIMSS and TIMSS Advanced, reveals patterns and trends culled from 20 years of data from Italy, Norway, the Russian Federation, Slovenia, and the United States. The report shows how misunderstandings can be traced back to inadequate foundational development of conceptual understanding in early grades. While this report focuses on gravity and linear equations, the general methodology based on item-level performance data described in the report can be applied to a range of topics from the different mathematics and science content domains covered in TIMSS and TIMSS Advanced. Policymakers and education systems can use the findings to identify specific needs in curriculum and instruction across grades.
Tad Johnston, AIR instructional coach, discussed why and how educators can use the report findings.
Q. Why is it important for educators to understand student misconceptions, errors, and misunderstandings like those highlighted in the AIR research?
Johnston: High-quality assessments force textbook writers, curriculum developers, professional developers, and educators to look at student performance to see if our kids are learning what they ought to be learning. AIR researchers’ analysis using TIMSS data helps us better understand specific student misconceptions, errors, and misunderstandings. We need to address the deficits students have or they’re not going to make it through higher-level coursework.
Some types of errors and misconceptions persist across the grades, so recognizing them early on gives educators a chance to address them. Teachers in the lower grades need to understand the higher-level concepts that kids are going to need when they get to middle school and high school.
Q. What do science educators need to know about the findings?
Johnston: In general, the TIMSS science items are conceptual, in that students are asked to provide descriptions, explanations, or predictions about phenomena with no need for computation or working with scientific formulas and symbols.
With physics, many misconceptions come from life experience—and incomplete observations or explanations of phenomena. For example, if I say a force is a “push” or “pull,” motion is involved in our minds. But as I sit still in my chair, gravity is exerting a force down on me and the chair is exerting a force up on me, but I don’t experience those forces. We can’t see them or feel them, or they’re counterintuitive. That’s why teachers need to work consciously to combat the misperceptions with lessons to address the contradictions in the way we perceive the world and the way the world really is. Exposing students at all grade levels to a broad range of problem-solving contexts provides opportunities to develop their level of understanding as well as confront common misconceptions.
For example, this TIMSS grade 8 physics sample item tests students’ understanding of gravity. Slightly more than one-third of students answered correctly that gravity acts on the jumper at all positions. The incorrect answers illustrate a common misconception that gravity acts only on falling objects and not on objects that are at rest.
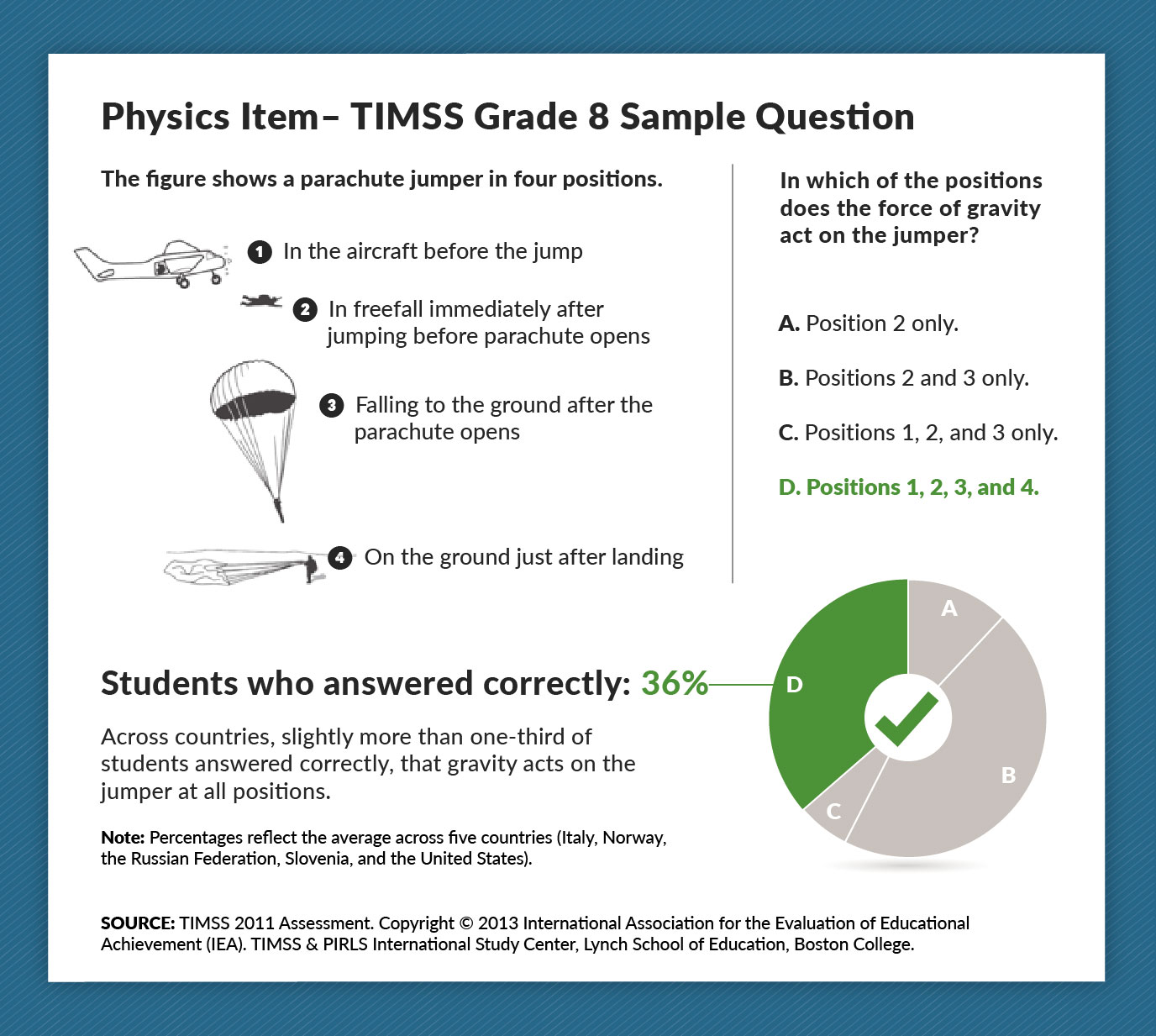
Q: How might teachers use this information to help their students understand the concept?
Johnston: Teachers could use this type of assessment item as a pretest for students to see what they think. Then they could structure some activities—and, importantly, conversations to go with the activities—to help students make sense of the activities and to address their misconceptions.
In upper elementary grades, teachers could talk about gravity being a force that pulls everything down to the surface of earth—when students sit in a chair, gravity is still pulling down on them and the chair is pushing up. The forces are balanced so they don’t move. In middle school, teachers could have students throw a ball up and down and then talk about how the constant force of gravity acting on the ball relates to the observed motion—gravity is acting on the ball at all times, even when it is traveling upward or not moving at all. By high school, students should be ready to apply Newton’s laws of motion to solve problems involving the constant acceleration due to the force of gravity. Teachers at this level could evaluate students’ understanding of gravitational force by using items such as the example shown here to address any lingering misconceptions before moving on to more advanced subject matter.
Q. And what should educators know about student errors in mathematics?
Johnston: Students use a variety of methods to solve math problems. For example, this TIMSS grade 8 algebra sample item requires students to apply their understanding of linear relationships to solve a contextual problem and show their work to support their answer. Though seemingly simple, the problem is complex, and only one-in-four students answered correctly with work shown. Students must apply a system of two relationships, the relative cost of a pen and a pencil and the combined cost of 2 pens and 3 pencils. The sample correct response on the left illustrates the application of an important algebraic idea—the substitution principle—where one variable is expressed in terms of another variable.
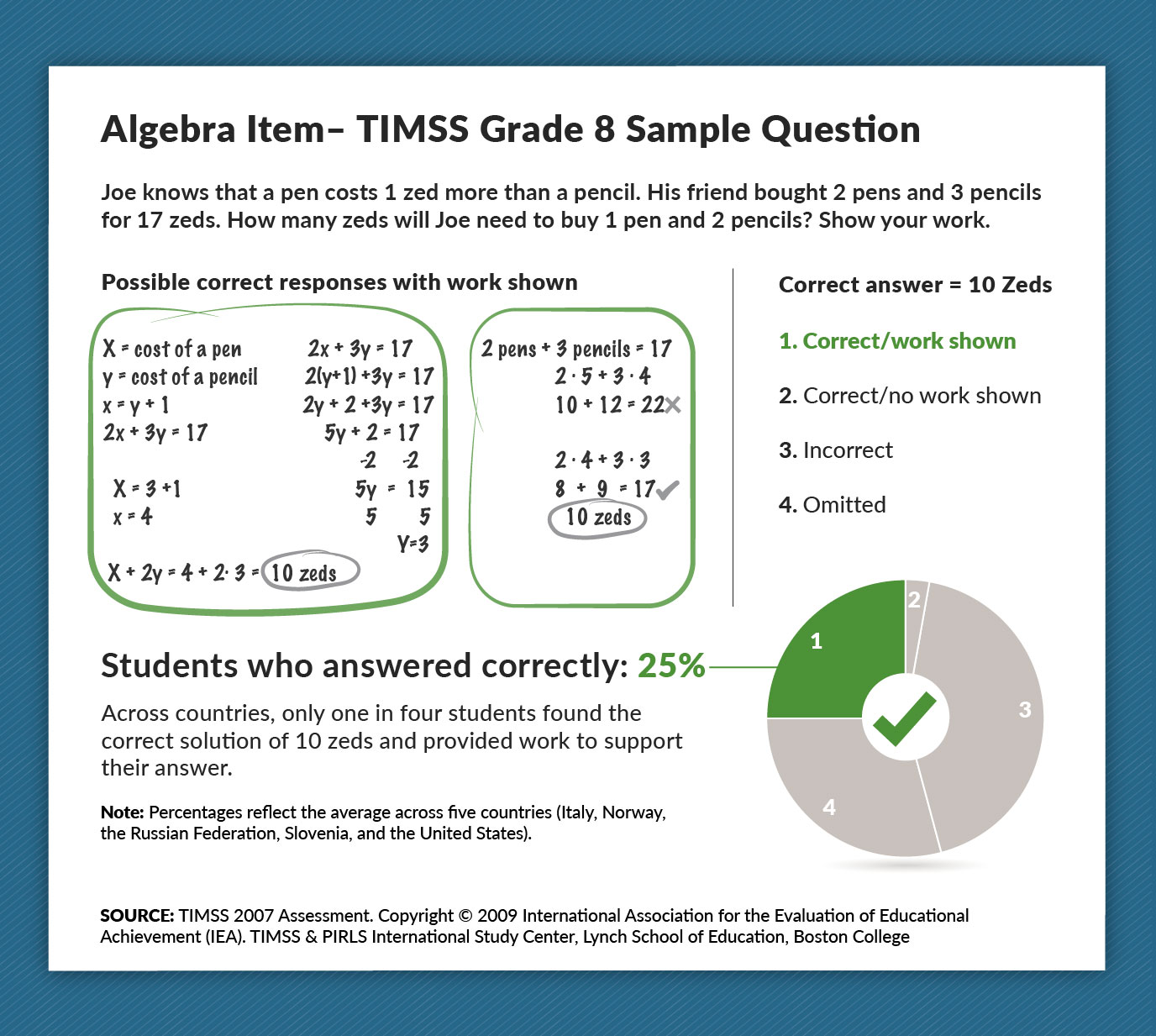
Interestingly, the majority of students who answered correctly did so without using equations and variables. It could be that the question as designed—using small numbers and whole number values—allowed students to use numerical methods, such as the sample response on the right, rather than formal algebra. It is also possible that many students had not yet been exposed to or were not confident in the use of systems of equations.
Q: How might mathematics instructors use this information in the classroom?
Johnston: It is hard to tell from the TIMSS reports on most mathematics items which student misconceptions are widespread or what types of errors were made. There are multiple ways for errors to occur on mathematics items, including errors in interpreting the scenarios, procedural errors (“Am I doing the steps right?”), conceptual errors (“I’m not doing the right steps”), or computational errors (“I calculated 12 times 7 is 72 instead of 84”).
Teachers in classrooms can do something that you can’t do on large-scale assessments—interview students. They can ask students why they selected a particular option on a multiple-choice item or ask them to explain how they solved a problem for open-ended items. Teachers can also analyze student work and ask, “What are the possible reasons for these errors?” and then plan an activity that can confront or dispel that thinking.
Algebraic thinking begins in elementary grades. Students describe relationships between sets of numbers and use arithmetic and other informal methods to solve problems involving unknown values. Gradually, students transition to more formal methods, but many students have difficulty translating problem-solving situations into equations and need more practice with this. Instead of using practice sheets that focus on solving equations out of context, students need more practice with word problems where they first write equations to model the problem situation and then solve the equations. Teachers can build on students’ informal methods to motivate the use and value of algebraic methods with equations. For example, teachers could begin a unit on systems of linear equations with a problem like the sample item and then show how formal methods are related to students’ informal thinking. Fluency with linear modeling and linear systems prepares students to work with more complex situations and mathematics.
Q. What else should educators consider?
Johnston: In the U.S., many state departments of education publish teaching ideas based on released assessment items. Virginia is one state that sends guidance on items that kids in the state are having difficulty with, so instructional coaches and teachers are informed about what they need to work on. Released items from national and international assessments such as TIMSS provide additional resources for science and mathematics educators to identify misunderstandings, develop pre-assessments, and provide focused instruction for their students.
A couple more things to think about: Are these types of misconceptions and errors addressed in curriculum materials that teachers have? And another issue is that some misconceptions are very persistent even among teachers—and we may not realize we have them.


